notneimanmarcus

Latest Posts by notneimanmarcus

Concorde’s cockpit.

Convair F2Y Sea Dart seaplane at rest on the water

Vic Elford Porsche Targa Florio 1969

@libertymints The Gee Bee was the perfect example of sticking the biggest motor you could on a brick.

P-61 Black Widow cockpit.

This Day in Aviation History

August 22nd, 1952

First flight of the Saunders-Roe Princess flying boat.

The Saunders-Roe SR.45 Princess was a British flying boat aircraft built by Saunders-Roe, based in Cowes on the Isle of Wight. The Princess was the largest all-metal flying boat ever constructed.

The project was cancelled after having produced only three examples. By the 1950s, large, commercial flying boats were being overshadowed by land-based aircraft. Factors such as runway and airport improvements added to the viability of land-based aircraft, which did not have the weight and drag of the boat hulls on seaplanes nor the issues with seawater corrosion.

The three airframes were stored against possible purchase but when an offer was made it was found that corrosion had set in; as a result they were scrapped….

Source:

Wikipedia, Saunders-Roe Princess: http://gstv.us/1MDH8iU  

YouTube, Saunders-Roe Princess Flying Boats: http://gstv.us/1MDH9U9  

If you enjoy the “This Day in Aviation History” collection, you may enjoy some of these other collections from Gazing Skyward TV: http://gstv.us/GSTVcollections  

Photo from: http://gstv.us/2b2abBr  

#avgeek #flying #boat #SaundersRoe #Princess #British #aviation #history

@jennisbaum

#TellAJokeDay….As you wish

https://wolfr.am/f0d8tarc

i feel like if at some point in your life you feel the need to say “a sheaf of e infinity rings on a moduli stack”, maybe something went wrong along the way

Illustrations from John Stillwell’s Classical Topology and Combinatorial Group Theory

Lagrange's theorem

Theorem: The size of a subgroup of finite group is divisible by the group’s size. That is, if H is a subgroup of G, |H| is a divisor of |G|.

Proof: Let’s start by saying we have a group G and a subgroup H.

This proof will count cosets. Specifically, I’ll use left cosets, but right cosets work the same way. Also, this proof will rely on a few properties of the integers.

I’ll prove this through lemmas, which are theorems used to prove other theorems. The distinction between a lemma and a theorem is only based on how we use them, and so historical reasons might leave some theorems as “lemmas.”

Keep reading

So, as a lot of you may know from following me, I’m the founder of a flying club at my university.

The main goal of this flying club is to give aviation opportunities to people who would otherwise not be able to afford it/be involved. (We are also an engineering club and all of our engineering projects are working towards this same goal of accessibility of aviation).

We are doing fundraisers. This is a picture of some demo envelopes that me and my club members made out of expired sectional charts. We want to sell them in order to make money for our club (to be spent on our engineering projects and outreach projects).

What do you guys think? I have no idea how to price them, so if anyone has input on what THEY would pay for them I’d be happy to hear it.

Thanks!

No road is long with good company.

Turkish Proverb (via fyp-philosophy)

Visual Representation of a Fourier transform whoaaaa hyo

Half Of The United States Lives In These Counties

In the creative mathematics, the role of proof is in no way restricted to its function of carrier of conviction. Otherwise, there would be no need for Carl Friedrich Gauss to consider eight (!) different proofs of the law of quadratic reciprocity. One metaphor of proof is a route, which might be a desert track boring and unimpressive until one finally reaches the oasis of ones destination, or a foot path in green hills, exciting and energizing, opening great vistas of unexplored lands and seductive offshoots, leading far away even after the initial destination point has been reached.

Yuri Manin, Foundations as Superstructure (Reflections of a practicing mathematician)

Minimalist Posters of Great Mathematicians.

how do you feel about tau

τ is cool, but really not practical for me. 

And I honestly do not feel like the τ vs π argument makes any sense at all. The two numbers are both technically correct and are just different ways of relating the circumference of a circle to two related, but DIFFERENT aspects of its geometry. 

As far as learning goes, τ does make a lot more intuitive sense than π and I will definitely give it that. It most certainly does make sense to relate the radius of a circle to its circumference! 

But the idea that π is wrong and that τ is somehow “more correct” is a weird logical fallacy:

There is a relation between the diameter of a circle and the circumference called π

There is a relation between the radius of a circle called τ

We use the measurements utilizing radii more than we use diameters

Therefore π is wrong. 

???? This argument doesn’t make sense. They’re two different relationships based on two different things. It’s like saying Fahrenheit is wrong because Celsius is easier to use.    

From the stance of someone in astrophysics, I really don’t think making τ the standard is practical at all. I have issues with it because τ is used as a symbol for many many MANY other things in my field. We use τ to represent time constants, optical depths, and torque and I feel like making the switch would be incredibly difficult and confusing because we use π in some of those. It’d make my life (and a bunch of other peoples’ lives) a lot more difficult to make the big shift, especially since π is not technically incorrect given its definition.

I say that both of them are good. I’m in camp “using π” because it’s most practical for me, but I don’t care if someone else uses τ because it works for them! 

Ok I got distracted but anyways yes this is my opinion

A picture from the un-official German Grand Prix held at the Solitudering, Stuttgart, in 1964. Jim Clark was the winner.

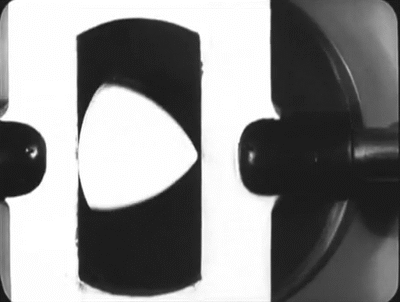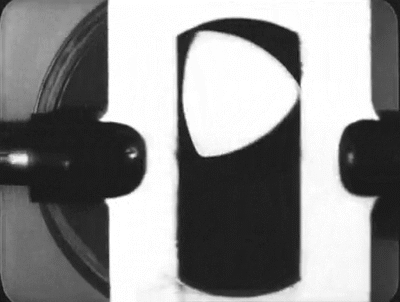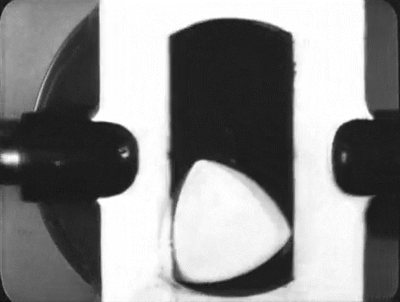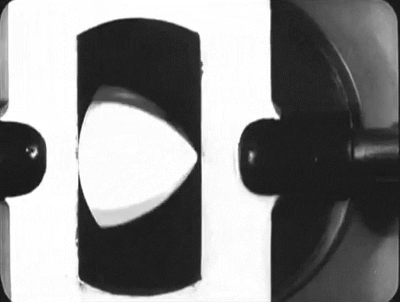
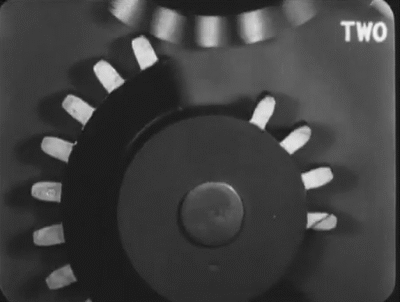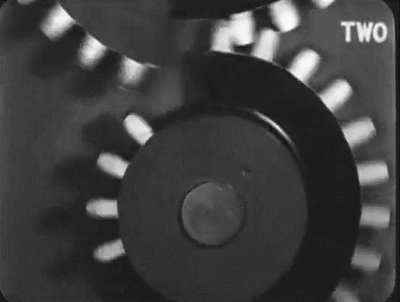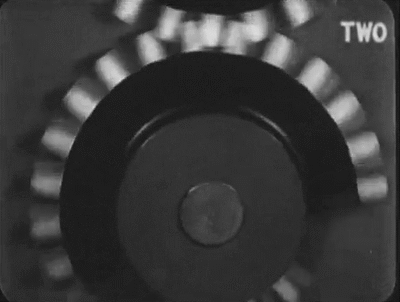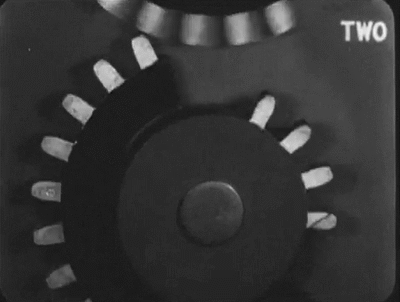
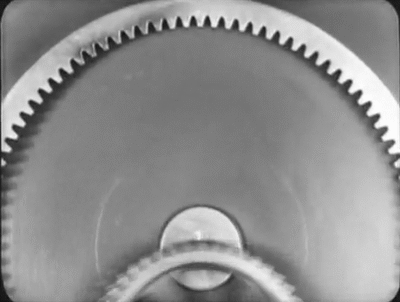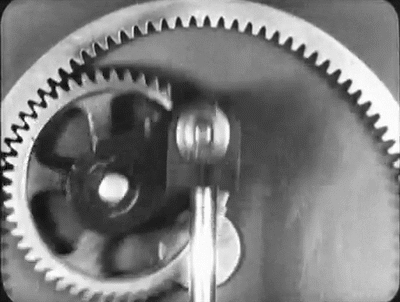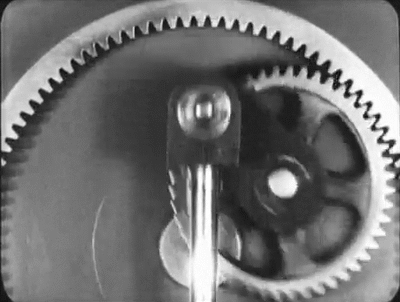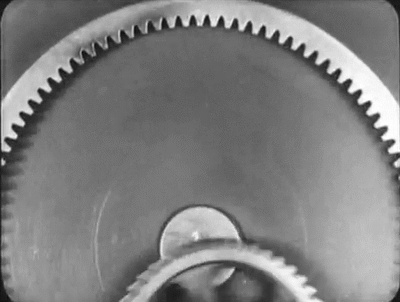
Mechanical Principles by Ralph Steiner (1930)

Dog Mountain- Washington

May 2013

So the universe is not quite as you thought it was. You’d better rearrange your beliefs, then. Because you certainly can’t rearrange the universe.

Isaac Asimov (via wordsnquotes)

کی

On the back roads of Glacier National Park, Montana With Andrea

The relationship between sine and cosine.

If you like this check out “How a Fourier series approximates a square wave.”

as seen here in katherines and paper towns, you are very opinionated on subdivisions. what are your feelings about them and why?

I really hated subdivisions as a teenager because to me they represented sameness and the bloated, intellectually disengaged, wretchedly average 21st century America.

I felt like all these identical houses were architectural crimes committed against the land, and like we would pay for our crimes with these stretched out, sprawling cities that human beings of the future would see as proof of the insanity that accompanied our national prosperity.

Now I live in that very suburbia. So….yeah.

The traditional Le Mans-start of the 12 Hours of Sebring, 1964.

Iceland’s sublime silence

A Canadian photographer turns her fascination with Iceland into a series celebrating the country’s raw and wondrous landscape