People Think Coding / Debugging Means Highly Concentrated Furious Typing, But Mostly It’s Just Angrily
People think coding / debugging means highly concentrated furious typing, but mostly it’s just angrily staring at the screen for long periods of time waiting for the problem to solve itself
More Posts from Jupyterjones and Others

Co-authored by physicists Ben Tippett* and David Tsang (non-fictional physicists at the fictional Gallifrey Polytechnic Institute and Gallifrey Institute of Technology, respectively), the paper – which you can access free of charge over on arXiv – presents a spacetime geometry that would make retrograde time travel possible. Such a spacetime geometry, write the researchers, would emulate “what a layperson would describe as a time machine“ [x]



#beingacomputerprogrammingmajor : Testing out html scripts on Pokemon Go and finding out it actually works.



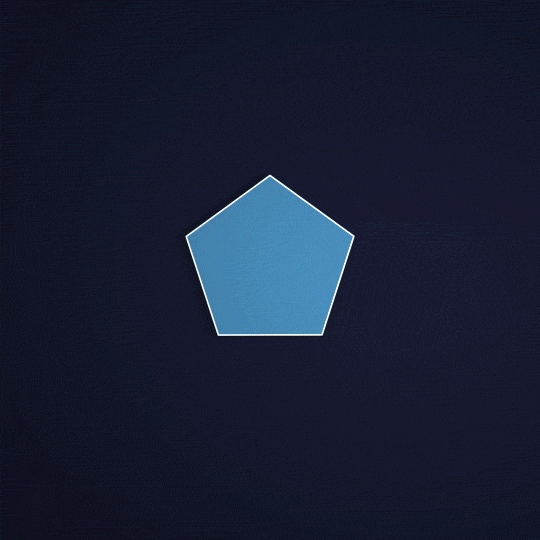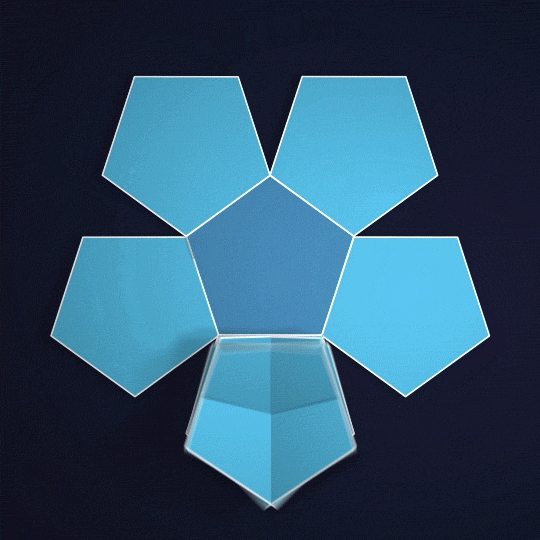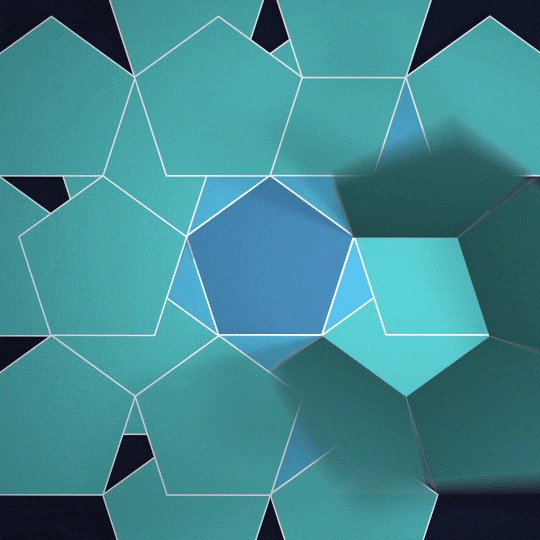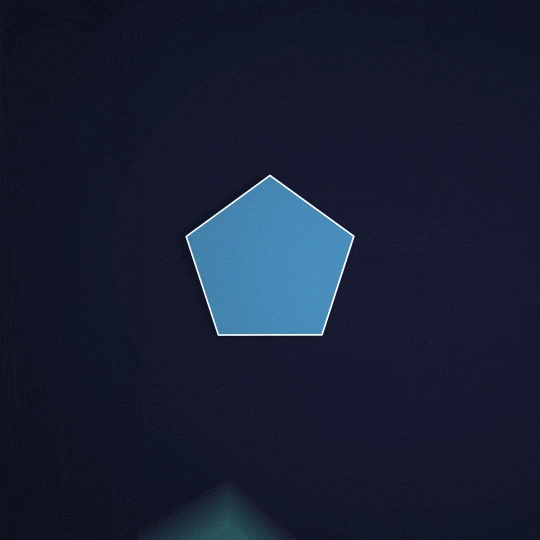

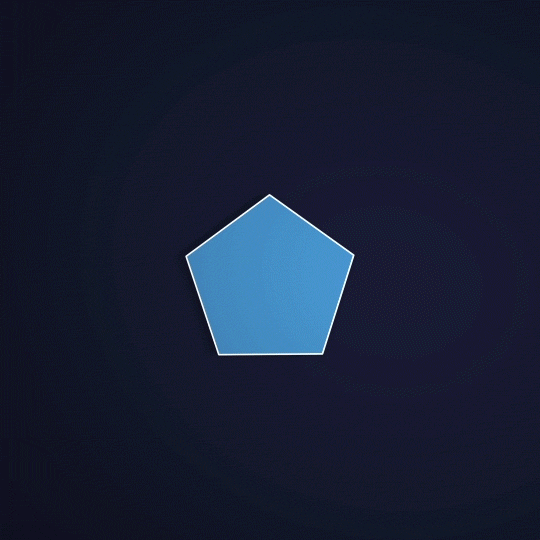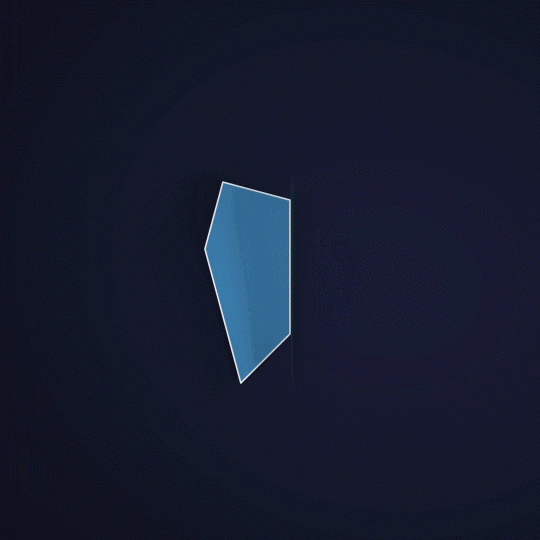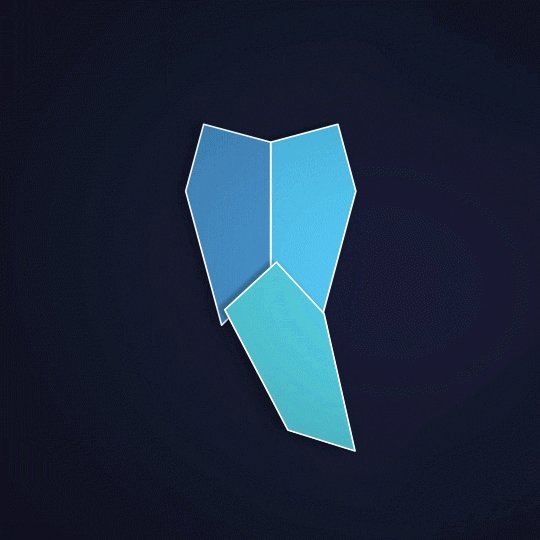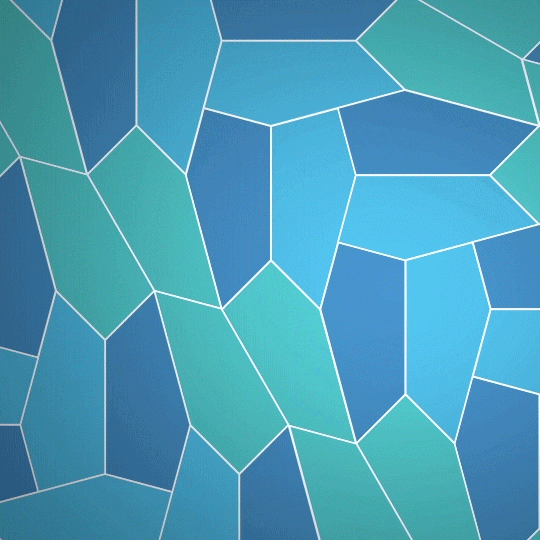
Big math news! It’s been thirty years since mathematicians last found a convex pentagon that could “tile the plane.” The latest discovery (by Jennifer McLoud-Mann, Casey Mann, and David Von Derau) was published earlier this month. Full story.
The Kakeya Problem
Some time ago I (briefly) mentioned that, along with two other students, I was taking a reading course this semester with Dmitriy Bilyk. It hasn’t quite gone in the direction we were initially expecting, but one of our long detours has been an extended sequence of readings around the the Kakeya conjecture. As far as I know, the Kakeya problem (different from the Kakeya conjecture; more on that later) was the first question that fell respectably under the purview of geometric measure theory. So if nothing else, it is interesting from a historical perspective as a question that kickstarted a whole new field of mathematics.
Okay, but what is the Kakeya problem?
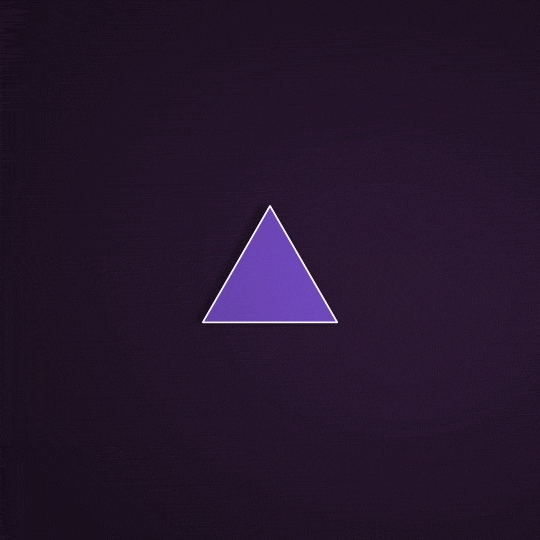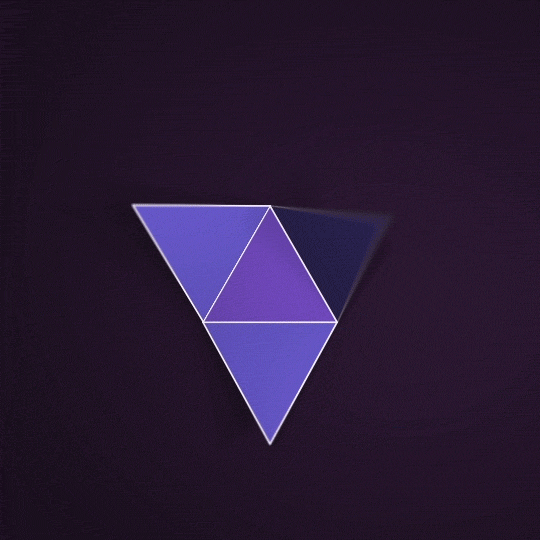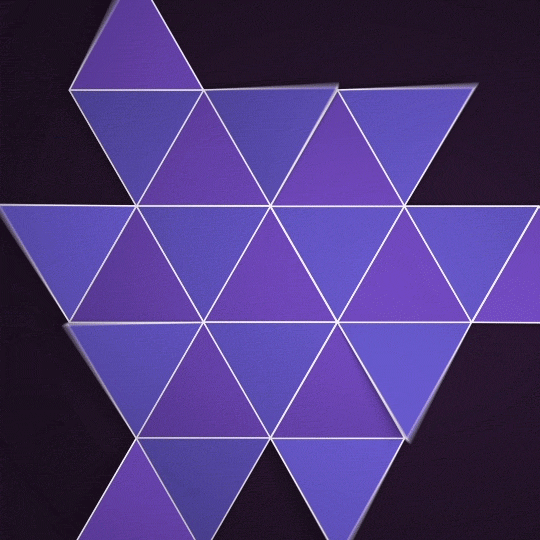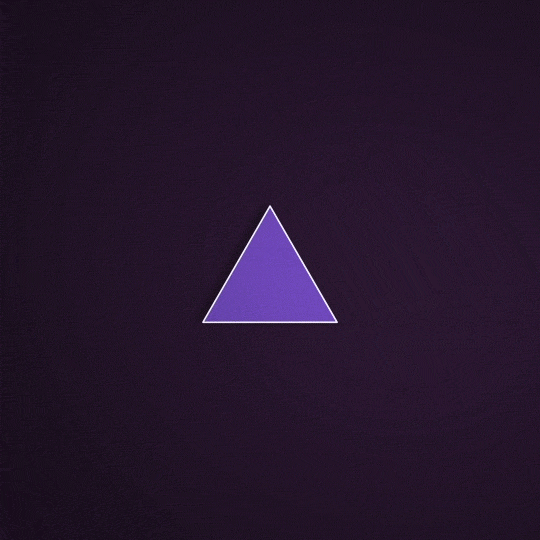
As originally posed, it goes like this: given a disk with diameter 1, it is possible to place down a line segment of length 1 into the set, and rotate it (continuously) an entire 180 degrees. But this is not the smallest-area set for which this kind of rotation is possible:

(all pictures in the post are from the Wikipedia page, which is really good)
This set has area $\tau/16$, half that of the circle. So the question naturally becomes: what is the area of the smallest set that allows this kind of rotation?
The answer is known, and it is…
…
zero, basically.
It’s remarkable, but it’s true: you can construct arbitrarily small sets in which you can perform a 180-degree rotation of a line segment! One way to do it goes like this: in the picture above, we reduced the area of the circle by squeezing it until it developed three points. If we keep squeezing to get more points, then the solid “middle” becomes very small, and the tendrils get very thin, so the area keeps decreasing. However, we can still take a line segment and slowly, methodically, shift it back and forth between the set’s pointy bits, parallel-parking style, to eventually get the entire 180 degrees of rotation.
——
You can’t actually get a set with zero area to work. But the reason for that seemed more like a technicality than something actually substantial. So people changed the problem slightly to get rid of those concerns. Now, instead of trying to get a set where you can rotate a line segment through all 180 degrees, you just have to have a set where you can find a line segment in every direction. The difference being that you don’t need to guarantee any smooth way to “move between” these line segments.
Sets that work for the modified problem are often called Kakeya sets (although some people reserve that for the rotation problem and use Besicovitch sets for the modified one). And indeed, there are Kakeya sets which actually have zero area.
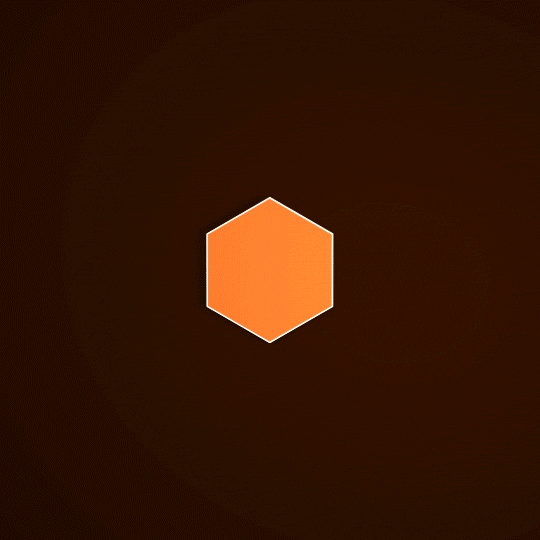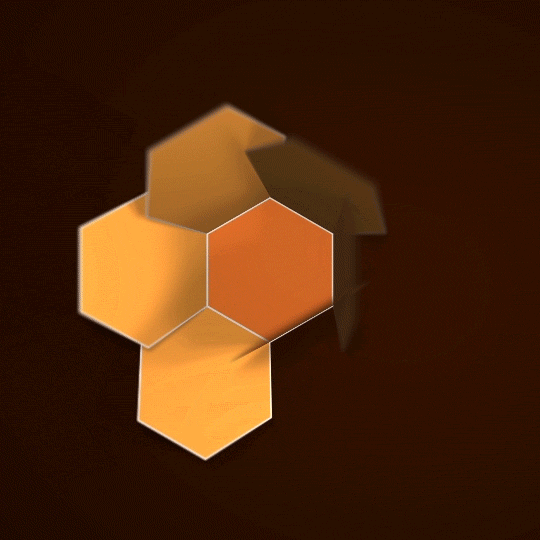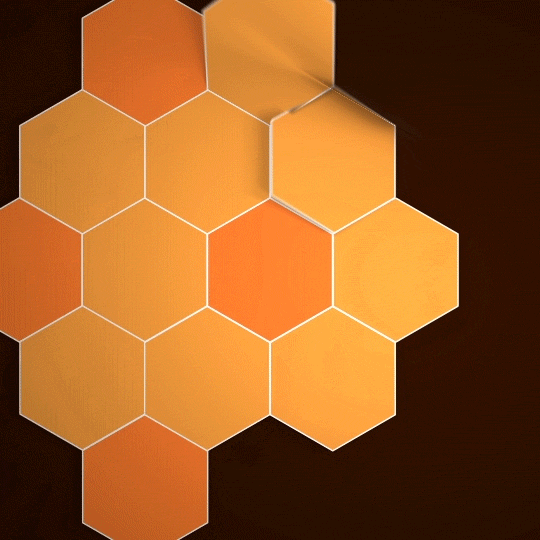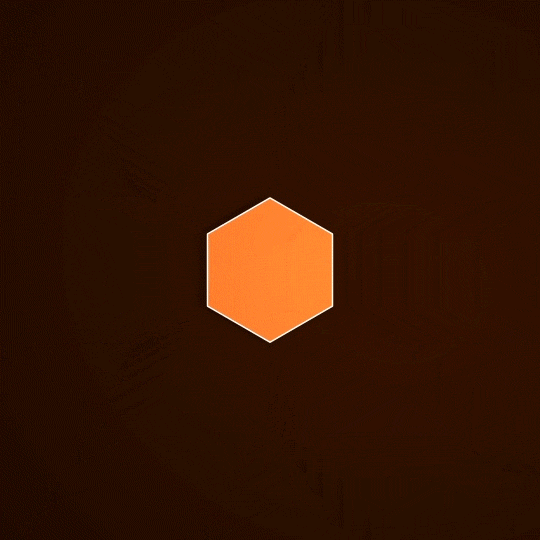
The details involved with going from “arbitrarily small” to “actually zero” are considerable, and we won’t get into them here. The following is a simplification (due to Perron) of Besicovitch’s original construction for the “arbitrarily small” case. We take a triangle which clearly has ½ of the directions the needle might possibly take, and split it up into several pieces in such a way that no directions are lost. Then we start to overlap those pieces to get a set (that still has segments in all the same directions) with much smaller area:

We can do this type of construction twice (one triangle “downward-facing” and the other “left-facing”) and then put those two sets together, guarantee that we get all of 180 degrees of directions.
This Besicovitch-Perron construction, itself, only produces sets which are “arbitrarily small”, but was later refined to go all the way to zero. Again, the technicalities involved in closing that gap are (much) more than I want to talk about now. But the fact that these technicalities can be carried out with the Besicovitch-Perron construction is what makes it “better” than the usual constructions for the original Kakeya problem.
——
I should conclude with a few words about the Kakeya conjecture, since I promised them earlier :)
Despite the essentially-solved status of these two classical Kakeya problems, there is at least one big question still left open. It is rather more technical than the original ones, and so doesn’t get a lot of same attention, but I’d like to take a stab at explaining what’s still current research in this sphere of ideas.
Despite the fact that Kakeya sets can be made to be “small” in the sense of measure, we still intuitively want to believe these sets are “big”. There are many ways we can formalize largeness of sets (in $\Bbb R^n$, in particular) but the one that seems to be most interesting for Kakeya things is the notion of Hausdorff dimension. I won’t define the term here, but if you’ve ever heard someone spouting off about fractals, you’ve probably heard the phrase “Fractals have non-integer dimension!”. This is the notion of dimension they’re talking about.
It is known that Kakeya sets in the plane have Hausdorff dimension 2, and that in general a Kakeya set in $n>2$ dimensions has Hausdorff dimension at least $\frac{n}{2}+1$. The proofs of these statements are… difficult, and the general case remains elusive.
One thing more: you can also formulate the Kakeya conjecture in finite fields: in this setting having “dimension $n$” in a vector space over the field $\Bbb F_q$ means that you have a constant times $q^n$ number of points in your set. Wolff proposed this “technicality-free” version in 1999 as a way to study the conjecture for $\Bbb R$. And indeed, a lot of the best ideas for the problem in $\Bbb R$ have come from doing some harmonic analysis on the ideas originally generated for the finite field case.
But then in 2008 Zeev Dvir went and solved the finite field case completely. Which on one hand is great! But on the other hand, Dvir’s method definitely can’t be finessed to work in $\Bbb R$ so we still have work to do :P
——
Partially I wanted to write about this because it’s cool in its own right, but I must admit that my main motivation is a little more pragmatic. There was a talk at SEICCGTC 2017 which showed a surprising connection between the Kakeya problem and a certain combinatorial game. So if you think these ideas are at all interesting, you may enjoy reading the next two posts in this sequence about that talk.
[ Post 1 ] [ Next ]

Programmer LoGiC


Jupiter’s Great Red Spot
Jupiter’s Great Red Spot (GRS) is an atmospheric storm that has been raging in Jupiter’s southern Hemisphere for at least 400 years.
About 100 years ago, the storm covered over 40,000 km of the surface. It is currently about one half of that size and seems to be shrinking.
At the present rate that it is shrinking it could become circular by 2040. The GRS rotates counter-clockwise(anti-cyclonic) and makes a full rotation every six Earth days.
It is not known exactly what causes the Great Red Spot’s reddish color. The most popular theory, which is supported by laboratory experiments, holds that the color may be caused by complex organic molecules, red phosphorus, or other sulfur compounds.
The GRS is about two to three times larger than Earth. Winds at its oval edges can reach up to 425 mph (680 km/h)
Infrared data has indicated that the Great Red Spot is colder (and thus, higher in altitude) than most of the other clouds on the planet
Sources: http://www.universetoday.com/15163/jupiters-great-red-spot/ http://www.space.com/23708-jupiter-great-red-spot-longevity.html
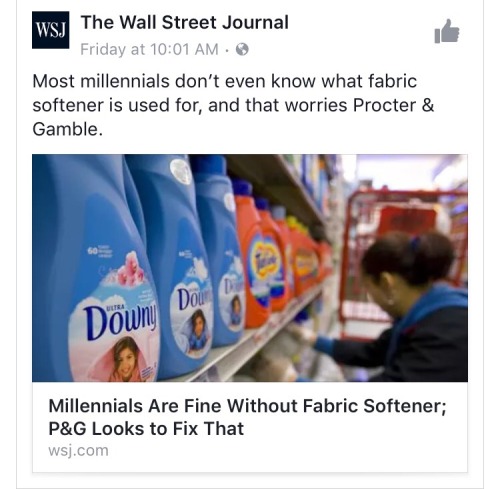
Weirdly anti-millennial articles have scraped the bottom of the barrel so hard that they are now two feet down into the topsoil
-
 lotus-ever-blooming liked this · 9 months ago
lotus-ever-blooming liked this · 9 months ago -
 h3lm1nth reblogged this · 1 year ago
h3lm1nth reblogged this · 1 year ago -
 grassepi liked this · 1 year ago
grassepi liked this · 1 year ago -
 writtenweaponry liked this · 2 years ago
writtenweaponry liked this · 2 years ago -
 stalker-ei reblogged this · 3 years ago
stalker-ei reblogged this · 3 years ago -
 treacherousgnome liked this · 3 years ago
treacherousgnome liked this · 3 years ago -
 ttttroublemaker reblogged this · 3 years ago
ttttroublemaker reblogged this · 3 years ago -
 chickenisamazing liked this · 4 years ago
chickenisamazing liked this · 4 years ago -
 randomwalkthroughcollege reblogged this · 4 years ago
randomwalkthroughcollege reblogged this · 4 years ago -
 urbanxfantasy liked this · 4 years ago
urbanxfantasy liked this · 4 years ago -
 thousandevilducks liked this · 4 years ago
thousandevilducks liked this · 4 years ago -
 outofdaydreams reblogged this · 4 years ago
outofdaydreams reblogged this · 4 years ago -
 sunflowersonthehill liked this · 5 years ago
sunflowersonthehill liked this · 5 years ago -
 coloress liked this · 5 years ago
coloress liked this · 5 years ago -
 fafik7 liked this · 5 years ago
fafik7 liked this · 5 years ago -
 likeshatteredrainbowglass liked this · 5 years ago
likeshatteredrainbowglass liked this · 5 years ago -
 pepperjellie reblogged this · 5 years ago
pepperjellie reblogged this · 5 years ago -
 thesinisterseventh liked this · 5 years ago
thesinisterseventh liked this · 5 years ago -
 peridarth reblogged this · 5 years ago
peridarth reblogged this · 5 years ago -
 gloxinian reblogged this · 5 years ago
gloxinian reblogged this · 5 years ago -
 aquariusxo14 liked this · 5 years ago
aquariusxo14 liked this · 5 years ago -
 wellhellojello liked this · 5 years ago
wellhellojello liked this · 5 years ago -
 theakwardsquid liked this · 6 years ago
theakwardsquid liked this · 6 years ago -
 culturedgiftedandblack-blog liked this · 6 years ago
culturedgiftedandblack-blog liked this · 6 years ago -
 enviy liked this · 6 years ago
enviy liked this · 6 years ago -
 full-of-awful-great-ideas liked this · 6 years ago
full-of-awful-great-ideas liked this · 6 years ago -
 code-cyber-punk-blog liked this · 6 years ago
code-cyber-punk-blog liked this · 6 years ago -
 dazzling-the-staging-environment liked this · 6 years ago
dazzling-the-staging-environment liked this · 6 years ago -
 softwareengineeringleader reblogged this · 6 years ago
softwareengineeringleader reblogged this · 6 years ago -
 libr-tumbl-alternative liked this · 6 years ago
libr-tumbl-alternative liked this · 6 years ago -
 forthosewhodontknow reblogged this · 6 years ago
forthosewhodontknow reblogged this · 6 years ago -
 bxbyporcelain liked this · 6 years ago
bxbyporcelain liked this · 6 years ago